Solving parity on 5×5 cube

Continue reading →

Dzień Matematyki w Radzyniu Podlaskim z Politechniką Lubelską

Dziękujemy za wspólne świętowanie Dnia Matematyki w Zespole Szkół Ponadgimnazjalnych w Radzyniu Podlaskim

[Pokaż miniatury]

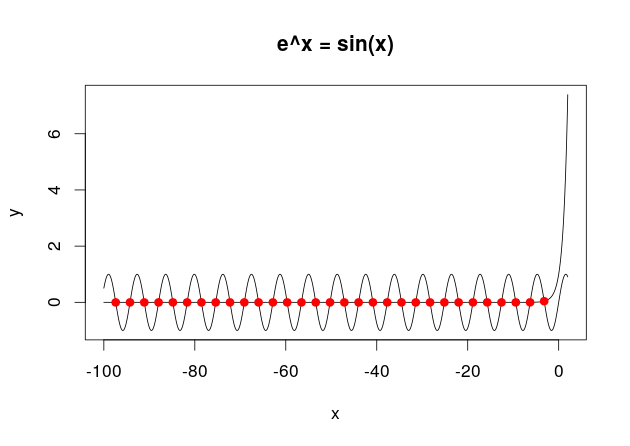
exp(x) = sin(x)

Some function
$f(x) = e^x-\sin(x)$
gives us interesting properties:

on the left side (x<0) we have $f(x) \approx \sin(x)$ and having infinitely many zeros
on the right side (x>0) we have $f(x) \approx e^x$ and growing exponentially

However, there is POI about zeros of this function:

Since $\sin(x) \approx x$ when x is small, we have approximation of first root as an solution of $e^x = - \pi - x$
$x_1 \approx - \pi - e^{-\pi - e^{-\pi - e^{-\pi - \ldots}}} = -W(e^{-\pi})-\pi \approx -3.18305$
where $W(x)$ is Lambert-W function.
The same way we have approximation of second root as an solution of $e^x = 2\pi + x$ $x_2 \approx - 2\pi + e^{-2\pi + e^{-2\pi + e^{-2\pi + \ldots}}} = -W(e^{-2\pi})-2\pi \approx -6.28131$
We know that $e^x \rightarrow 0$ as $x \rightarrow - \infty$ there is $x_n \rightarrow -n\pi$ , although we don’t know the exact solution yet..